SNOSB87D March 2011 – May 2019 LM21215A
PRODUCTION DATA.
- 1 Features
- 2 Applications
- 3 Description
- 4 Revision History
- 5 Pin Configuration and Functions
- 6 Specifications
- 7 Detailed Description
- 8 Application and Implementation
- 9 Power Supply Recommendations
- 10Layout
- 11Device and Documentation Support
- 12Mechanical, Packaging, and Orderable Information
Package Options
Mechanical Data (Package|Pins)
- PWP|20
Thermal pad, mechanical data (Package|Pins)
- PWP|20
Orderable Information
8.2.1.2.7 Control Loop Compensation
This section walks through the various steps in obtaining the open-loop transfer function. There are three main blocks of a voltage-mode buck converter that the power supply designer must consider when designing the control system: the power stage, the PWM modulator, and the compensated error amplifier. The control loop architecture of a voltage-mode buck converter is provided in Figure 32.
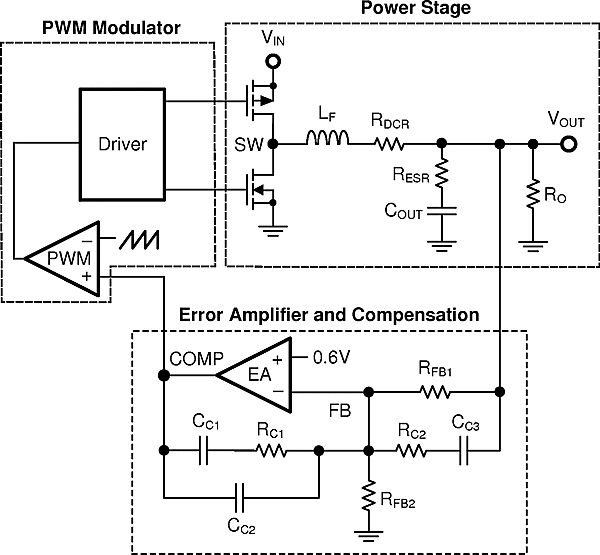 Figure 32. Voltage-mode Buck Converter Architecture
Figure 32. Voltage-mode Buck Converter Architecture The power stage consists of the filter inductor (LF) with DCR (DC resistance RDCR), output capacitor (COUT) with ESR (effective series resistance RESR), and load resistance (RO). The LM21215A incorporates a high-bandwidth error amplifier between the FB and COMP pins to achieve high loop bandwidth. The error amplifier (EA) constantly regulates FB to 0.6 V. The compensation component network around the error amplifier establish system stability. The modulator creates the duty cycle command by comparing the error amplifier output with an internally-generated PWM ramp set at the switching frequency.
There are three transfer functions that are taken into consideration when obtaining the total open-loop transfer function; COMP-to-duty cycle (modulator), duty cycle-to-VOUT (power stage), and VOUT-to-COMP (compensator). If ΔVRAMP is the peak-to-peak ramp voltage (nominally 0.8 V), the COMP-to-duty cycle transfer function is simply the PWM modulator gain given by Equation 10.
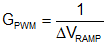
The duty cycle-to-output transfer function includes the filter inductor, output capacitor, and output load resistance. The inductor and capacitor create a pair of complex poles at the LC tank frequency expressed by Equation 11.
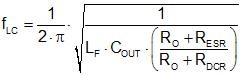
In addition to two complex poles, a left half plane zero is created by the output capacitor ESR located at a frequency described by Equation 12.
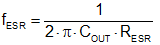
A Bode plot showing the –40dB/decade power stage response is shown in Figure 33
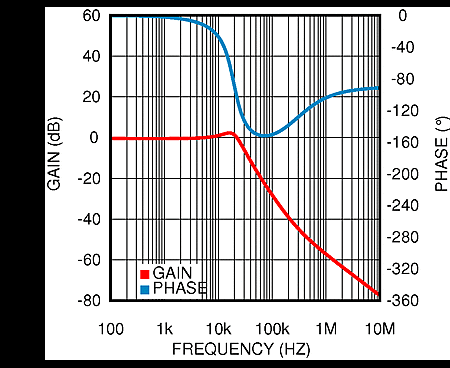 Figure 33. Power Stage Bode Plot
Figure 33. Power Stage Bode Plot The complex poles created by the filter inductor and output capacitor cause a 180° phase lag. The phase is boosted back up to –90° by the output capacitor ESR zero. The compensator must provide sufficient phase boost to stabilize the loop response. The type-III compensation network shown around the error amplifier in Figure 32 creates two poles, two zeros and a pole at the origin. Placing these poles and zeros at the correct frequencies stabilizes the closed loop response. The compensator transfer function is given by Equation 13.

where
- Kmid is the mid-band gain, RC1/RFB1
The pole located at the origin gives high open-loop gain at DC, translating into improved load regulation accuracy. This pole occurs at a very low frequency due to the finite gain of the error amplifier; however, its location is approximated at DC for the purposes of compensation. The other two poles and two zeros are located accordingly to stabilize the voltage-mode loop depending on the power stage complex poles and their quality factor, Q. Figure 34 illustrates a typical type-III compensator transfer function.
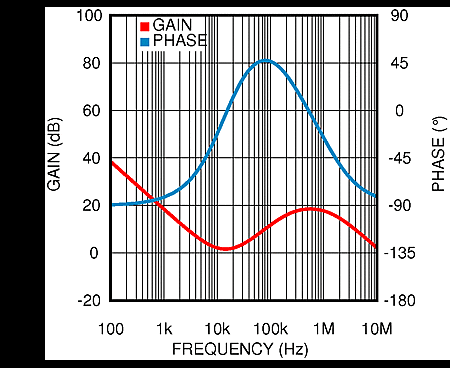 Figure 34. Type-III Compensation Network Bode Plot
Figure 34. Type-III Compensation Network Bode Plot As seen in Figure 34, the two compensator zeros located at (fLC/2, fLC) provide a phase boost. This mitigates the effect of the phase loss from the output filter. The compensation network also adds two poles to the system. One pole is located at the output capacitor ESR zero (fESR) and the other pole is at half the switching frequency (FSW/2) to roll off the high frequency response.
The dependency of the pole and zero locations on the compensation components is described as follows:
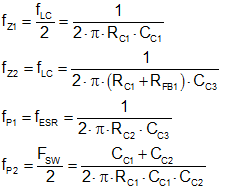
The output capacitance, COUT, depends on capacitor chemistry and bias voltage. For multi-layer ceramic capacitors (MLCC), the total capacitance degrades as the DC bias voltage is increased. To accurately calculate and optimize the compensation network, it is advisable to determine the effective capacitance of the output capacitors when biased at the output voltage.
The example given here is the total output capacitance using three MLCC output capacitors biased at 1.2 V, as seen in the typical application schematic of Figure 28. 50% capacitance derating is assumed.
NOTE
It is more conservative, from a stability standpoint, to err on the side of a lower output capacitance in the compensation calculations rather than a higher, as this will result in a lower bandwidth but increased phase margin.
First, choose a resistance for RFB1, a typical value being 10 kΩ. From this, calculate the resistance of RC1 using Equation 14 to set the mid-band gain such that the desired crossover frequency is achieved.
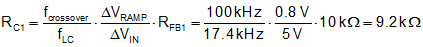
Next, calculate the capacitance of CC1 by placing a zero at half of the LC double pole frequency (fLC):

Now calculate CC2 to place a pole at half of the switching frequency and RC2 to place the second zero at the LC double pole frequency:
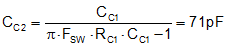
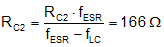
Last, derive capacitance of CC3 to place a pole at the same frequency as the output capacitor ESR zero:
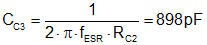
An illustration of the total loop response is seen in Figure 35.
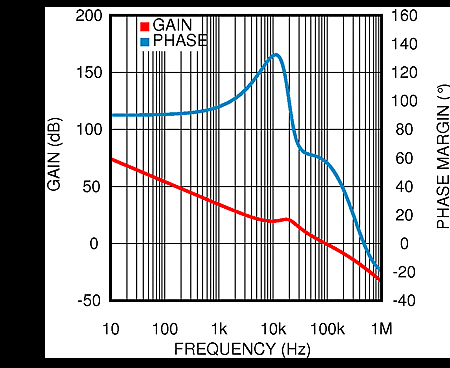 Figure 35. Loop Response
Figure 35. Loop Response It is important to verify the stability either by observing the load transient response or by using a network analyzer. A phase margin between 45° and 70° is usually desired for voltage-mode converter circuits. Excessive phase margin causes slow system response to load transients whereas low phase margin leads to an oscillatory load transient response. If the peak deviation of the load transient response is larger than required, increasing fcrossover and recalculating the compensation components may help but usually at the expense of phase margin.
Table 2. Bill of Materials (VIN = 3.3 V to 5.5 V, VOUT = 1.2 V, IOUT = 15 A, fSW = 500 kHz)
| REF DES | DESCRIPTION | VENDOR | PART NUMBER | QUANTITY |
|---|---|---|---|---|
| CF | CAP, CERM, 1 µF, 10 V, ±10%, X7R, 0603 | MuRata | GRM188R71A105KA61D | 1 |
| CIN1, CIN2, CIN3, CO1, CO2, CO3 | CAP, CERM, 100 µF, 6.3 V, ±20%, X5R, 1206 | MuRata | GRM31CR60J107ME39L | 6 |
| CC1 | CAP, CERM, 1800 pF, 50 V, ±5%, C0G/NP0, 0603 | TDK | C1608C0G1H182J | 1 |
| CC2 | CAP, CERM, 68 pF, 50 V, ±5%, C0G/NP0, 0603 | TDK | C1608C0G1H680J | 1 |
| CC3 | CAP, CERM, 820 pF, 50 V, ±5%, C0G/NP0, 0603 | TDK | C1608C0G1H821J | 1 |
| CSS | CAP, CERM, 0.033 µF, 16 V, ±10%, X7R, 0603 | MuRata | GRM188R71C333KA01D | 1 |
| LF | Inductor, Powdered Iron, 560 nH, 27.5A, 1.8 mΩ, SMD | Vishay Dale | IHLP4040DZERR56M01 | 1 |
| RF | RES, 1 Ω, 5%, 0.1 W, 0603 | Vishay Dale | CRCW06031R00JNEA | 1 |
| RC1 | RES, 9.31 kΩ, 1%, 0.1 W, 0603 | Vishay Dale | CRCW06039K31FKEA | 1 |
| RC2 | RES, 165 Ω, 1%, 0.1 W, 0603 | Vishay Dale | CRCW0603165RFKEA | 1 |
| RFB1, RFB2, RPGOOD | RES, 10 kΩ, 1%, 0.1 W, 0603 | Vishay Dale | CRCW060310K0FKEA | 3 |
| U1 | LM21215A Synchronous Buck Regulator | TI | LM21215AMH-1/NOPB | 1 |