SLOA011B January   2018  – July 2021 LF347 , LF353 , LM348 , MC1458 , TL022 , TL061 , TL062 , TL071 , TL072 , UA741

 

  1. 1Introduction
    1. 1.1 Amplifier Basics
    2. 1.2 Ideal Op Amp Model
  2. 2Non-Inverting Amplifier
    1. 2.1 Closed Loop Concepts and Simplifications
  3. 3Inverting Amplifier
    1. 3.1 Closed Loop Concepts and Simplifications
  4. 4Simplified Op Amp Circuit Diagram
    1. 4.1 Input Stage
    2. 4.2 Second Stage
    3. 4.3 Output Stage
  5. 5Op Amp Specifications
    1. 5.1  Absolute Maximum Ratings and Recommended Operating Condition
    2. 5.2  Input Offset Voltage
    3. 5.3  Input Current
    4. 5.4  Input Common Mode Voltage Range
    5. 5.5  Differential Input Voltage Range
    6. 5.6  Maximum Output Voltage Swing
    7. 5.7  Large Signal Differential Voltage Amplification
    8. 5.8  Input Parasitic Elements
      1. 5.8.1 Input Capacitance
      2. 5.8.2 Input Resistance
    9. 5.9  Output Impedance
    10. 5.10 Common-Mode Rejection Ratio
    11. 5.11 Supply Voltage Rejection Ratio
    12. 5.12 Supply Current
    13. 5.13 Slew Rate at Unity Gain
    14. 5.14 Equivalent Input Noise
    15. 5.15 Total Harmonic Distortion Plus Noise
    16. 5.16 Unity-Gain Bandwidth and Phase Margin
    17. 5.17 Settling Time
  6. 6References
  7. 7Glossary
  8. 8Revision History

2 Non-Inverting Amplifier

An ideal op amp by itself is not a very useful device since any finite input signal would result in infinite output. By connecting external components around the ideal op amp, we can construct useful amplifier circuits. Figure 2-1 shows a basic op amp circuit, the non-inverting amplifier. The triangular gain block symbol is used to represent an ideal op amp. The input terminal marked with a + (Vp) is called the non-inverting input; – (Vn) marks the inverting input.

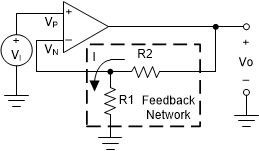GUID-3642E90B-8578-4895-98E8-BF3F1D28B4F9-low.gif Figure 2-1 Non-Inverting Amplifier

To understand this circuit we must derive a relationship between the input voltage, Vi, and the output voltage, Vo.

Remembering that there is no loading at the input,

Equation 11. Vp = VI

The voltage at Vn is derived from Vo via the resistor network, R1 and R2, so that,

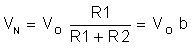
Equation 12. GUID-C03B9A99-9D7A-411A-8B09-BA49BEB97054-low.gif

where,

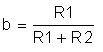
Equation 13. GUID-8FD775C9-C2FF-4068-AFBF-556DC71AF2E5-low.gif

The parameter b is called the feedback factor because it represents the portion of the output that is fed back to the input.

Recalling the ideal model,

Equation 14. Vo = aVσ = a(Vp - Vn)

Substituting,

Equation 15. Vo = a(Vi - bVo)

and collecting terms yield,

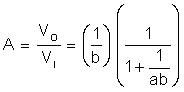
Equation 16. GUID-4B8F75BC-BF03-4205-8254-AE7C1DA96EAC-low.gif

This result shows that the op amp circuit of Figure 2-1 is itself an amplifier with gain A. Since the polarity of Vi and VO are the same, it is referred to as a non-inverting amplifier.

A is called the close loop gain of the op amp circuit, whereas a is the open loop gain. The product ab is called the loop gain. This is the gain a signal would see starting at the inverting input and traveling in a clockwise loop through the op amp and the feedback network.