TIDUC07 March   2022

 

  1.   Description
  2.   Resources
  3.   Features
  4.   Applications
  5.   5
  6. 1System Description
  7. 2System Overview
    1. 2.1 Block Diagram
    2. 2.2 Highlighted Products
      1. 2.2.1 TMAG5170
      2. 2.2.2 DRV5055A4
    3. 2.3 Design Considerations
      1. 2.3.1 Magnet Selection
      2. 2.3.2 Magnet Shape
      3. 2.3.3 Magnet Rotation Speed
      4. 2.3.4 Sensor Location
      5. 2.3.5 Expected Performance
      6. 2.3.6 Layout for Sensor Location
      7. 2.3.7 45° Alignment
  8. 3Hardware, Software, Testing Requirements, and Test Results
    1. 3.1 Hardware Requirements
    2. 3.2 Test Setup
      1. 3.2.1 Test Equipment
      2. 3.2.2 Test Hardware Configuration
      3. 3.2.3 Test Software Configuration and Initial Data Capture
    3. 3.3 Test Results
      1. 3.3.1 Calibration Methods
      2. 3.3.2 TMAG5170 On-Axis
      3. 3.3.3 TMAG5170 In-Plane
      4. 3.3.4 TMAG5170 Off-Axis
      5. 3.3.5 TMAG5170 45° Alignment
      6. 3.3.6 DRV5055 Off Axis Result
  9. 4Design and Documentation Support
    1. 4.1 Design Files
      1. 4.1.1 Schematics
      2. 4.1.2 BOM
    2. 4.2 Tools and Software
    3. 4.3 Documentation Support
    4. 4.4 Support Resources
    5. 4.5 Trademarks

2.3.7 45° Alignment

The TIDA-060040 also shows an additional alignment option for TMAG5170.

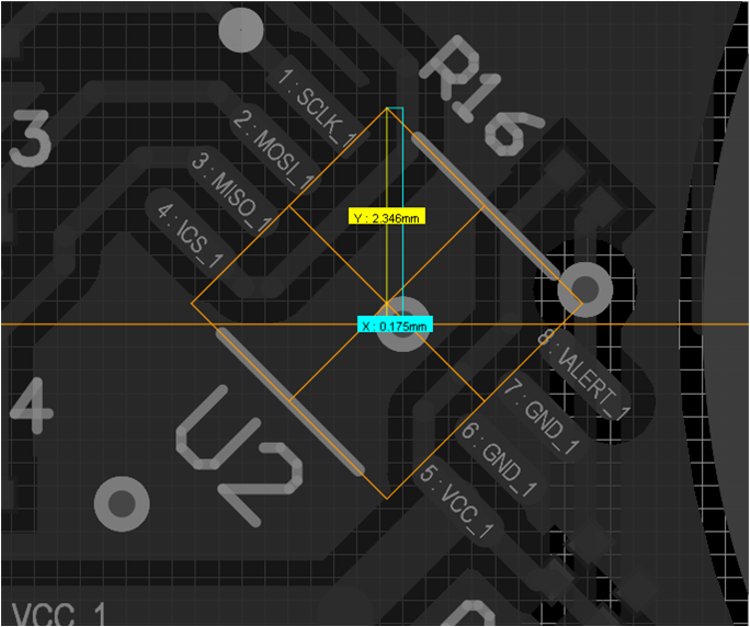GUID-20220208-SS0I-GRCX-VHPS-7TMX6FPSNPGQ-low.png Figure 2-23 TMAG5170 45° Alignment

This second sensor location option has the device physically rotated 45°. Instead of the normal orthogonal alignment, the X and Y axes of the sensor each observe the same input magnitude. The effective input for each axis is the average of the two axes. The benefit of installing the device with this rotation is that it allows the sensor to be placed in locations where one input might be excessively large, one input might not have sufficient SNR, or both. This alignment offers a potentially more compact solution, but at the cost of phase alignment. By rotating the sensor in this fashion, a phase error is introduced which is a function of the total mismatch between the two channels. This phase error is depicted as β in Equation 5. To properly use inputs with a phase error, the following trigonometric adjustment to the arctangent calculation is helpful:

Equation 5. A cos ⁡ θ - β = A cos ⁡ θ cos ⁡ β + A sin ⁡ θ sin ⁡ β
Equation 6. I n 2 I n 1 =   A cos ⁡ β cos ⁡ θ + A sin ⁡ θ sin ⁡ β A sin ⁡ θ
Equation 7. I n 2 I n 1 = cos ⁡ β tan ⁡ θ + sin ⁡ β
Equation 8. tan ⁡ θ =   cos ⁡ β I n 2 I n 1 - sin ⁡ β
Equation 9. θ = ⁡ t a n - 1   cos ⁡ β I n 2 I n 1 - sin ⁡ β

When applying Equation 9, it is important to verify the actual phase shift of the vector components. Manufacturing alignment errors and the combination of unequal amplitude inputs may result in a value of β which differs from the physical rotation of the sensor.