SLOS930C November 2015 – October 2024 THS4541-Q1
PRODUCTION DATA
- 1
- 1 Features
- 2 Applications
- 3 Description
- 4 Device Comparison Table
- 5 Pin Configuration and Functions
-
6 Specifications
- 6.1 Absolute Maximum Ratings
- 6.2 ESD Ratings
- 6.3 Recommended Operating Conditions
- 6.4 Thermal Information
- 6.5 Electrical Characteristics: (Vs+) – Vs– = 5 V
- 6.6 Electrical Characteristics: (Vs+) – Vs– = 3 V
- 6.7 Typical Characteristics: 5-V Single Supply
- 6.8 Typical Characteristics: 3-V Single Supply
- 6.9 Typical Characteristics: 3-V to 5-V Supply Range
-
7 Parameter Measurement Information
- 7.1 Example Characterization Circuits
- 7.2 Frequency-Response Shape Factors
- 7.3 I/O Headroom Considerations
- 7.4 Output DC Error and Drift Calculations and the Effect of Resistor Imbalances
- 7.5 Noise Analysis
- 7.6 Factors Influencing Harmonic Distortion
- 7.7 Driving Capacitive Loads
- 7.8 Thermal Analysis
-
8 Detailed Description
- 8.1 Overview
- 8.2 Functional Block Diagram
- 8.3 Feature Description
- 8.4
Device Functional Modes
- 8.4.1
Operation from Single-Ended Sources to Differential Outputs
- 8.4.1.1 AC-Coupled Signal Path Considerations for Single-Ended Input to Differential Output Conversion
- 8.4.1.2 DC-Coupled Input Signal Path Considerations for Single-Ended to Differential Conversion
- 8.4.1.3 Resistor Design Equations for the Single-Ended to Differential Configuration of the FDA
- 8.4.1.4 Input Impedance for the Single-Ended to Differential FDA Configuration
- 8.4.2 Differential-Input to Differential-Output Operation
- 8.4.1
Operation from Single-Ended Sources to Differential Outputs
- 9 Application and Implementation
- 10Device and Documentation Support
- 11Revision History
- 12Mechanical, Packaging, and Orderable Information
Package Options
Refer to the PDF data sheet for device specific package drawings
Mechanical Data (Package|Pins)
- RGT|16
Thermal pad, mechanical data (Package|Pins)
- RGT|16
Orderable Information
9.2.1.2 Detailed Design Procedure
Operating the THS4541-Q1 at a low dc noise gain, or with higher feedback resistors, can cause a lower phase margin to exist, giving the response peaking shown in Figure 6-1 for the gain of 0.1 (a 1/10 attenuator) condition. Although operating the THS4541-Q1 as an attenuator (taking a large input range to a purely differential signal around a controlled-output, common-mode voltage) is often useful, the response peaking illustrated in Figure 6-1 is usually undesirable. Several methods can be used to reduce or eliminate this peaking; usually, at the cost of higher output noise. Using dc techniques always increases the output noise broadband, while using an ac noise-gain-shaping technique peaks the noise, but only at higher frequencies that can then be filtered off with the typical passive filters often used after this stage. Figure 9-1 shows a simplified schematic for the gain of 0.1 V/V test from Figure 7-1.
This configuration shows a nominal 18° phase margin (from Table 7-2); therefore, a very highly-peaked response is illustrated in Figure 6-1. This peaking can be eliminated by placing two feedback capacitors across the Rf elements and a differential input capacitor. Adding these capacitors provides a transition from a resistively set noise gain (NG1 here; 1.1 in Table 7-2) to a capacitive divider at high-frequency flattening out to a higher noise gain (NG2 here). The key for this approach is to target a Zo, where the noise gain begins to peak up. Using only the following terms, and targeting a closed-loop flat (Butterworth) response, gives this solution sequence for Zo and then the capacitor values.
- Gain bandwidth product in Hz (850 MHz for the THS4541-Q1)
- Low frequency noise gain, NG1 ( = 1.1 in the attenuator gain of 0.1 V/V design)
- Target high-frequency noise gain selected to be higher than NG1 (NG2 = 3.1 V/V is selected for this design)
- Feedback resistor value, Rf (assumed balanced for this differential design = 402 Ω for this design example)
From these elements, for any decompensated voltage-feedback op amp or FDA, solve for Zo (in Hz) using Equation 15:
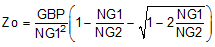
From this target zero frequency in the noise gain, solve for the feedback capacitors using Equation 16:

The next step is to resolve the input capacitance on the summing junction. Equation 17 is for a single-ended op amp (for example, OPA847) where that capacitor goes to ground. To use Equation 17 for a voltage-feedback FDA, cut the target value in half, and place the result across the two inputs (reducing the external value by the specified internal differential capacitance).

Setting the external compensation elements using Equation 15 to Equation 17 allows an estimate of the resulting flat bandwidth f–3dB frequency, as shown in Equation 18:
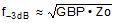
Running through these steps for the THS4541-Q1 in the attenuator circuit of Figure 9-1 gives the proposed compensation of Figure 9-2 where Equation 18 estimates a bandwidth of 252 MHz (Zo target is 74.7 MHz).
 Figure 9-2 Compensated Attenuator
Circuit Using the THS4541-Q1
Figure 9-2 Compensated Attenuator
Circuit Using the THS4541-Q1The 1 pF across the inputs is really a total 1.85 pF, including the internal differential capacitance, and a Cs = 3.7 pF for a single-ended design from Equation 17.
These two designs (with and without the capacitors) were both bench tested and simulated using the THS4541 TINA model giving the results of Figure 9-3.
This method does a good job of flattening the response for what starts out as a low phase-margin attenuator application. The simulation model does a very good job of predicting the peaking and showing the same improvement with the external capacitors; both giving a flat, approximately 250-MHz, closed-loop bandwidth for this gain of a 0.1-V/V design. In this example, the output noise begins to peak up (as a result of the noise-gain shaping of the capacitors) above 70 MHz. Use postfiltering to minimize any increase in the integrated noise using this technique. Using this solution to deliver an 8-VPP differential output to a successive approximation register (SAR) ADC (using the 2.5-V Vocm shown), the circuit accepts up to ±40-V inputs, where the 4-kΩ input Rg1 draws ±10 mA from the source.