-
Understanding Op Amp Noise in Audio Circuits SBOA345 June 2021 ADC10D1000QML-SP , ADC12D1600QML-SP , ADC12D1620QML-SP , INA1620 , OPA132 , OPA134 , OPA1602 , OPA1604 , OPA1611 , OPA1612 , OPA1622 , OPA1632 , OPA1637 , OPA1641 , OPA1642 , OPA1644 , OPA1652 , OPA1654 , OPA1655 , OPA1656 , OPA1662 , OPA1664 , OPA1671 , OPA1677 , OPA1678 , OPA1679 , OPA1688 , OPA1692 , OPA2132 , OPA2134 , OPA4132 , OPA4134
Understanding Op Amp Noise in Audio Circuits
Trademarks
All trademarks are the property of their respective owners.
1 Thermal Noise
Resistors can be a major contribution to the overall noise of an audio circuit. The noise generated from a resistor, also known as thermal noise, is noise generated by the random motion of charges within the resistor. We can calculate the noise generated by an ideal resistor using Equation 1.
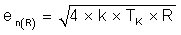
Where
- en(R) = Noise spectral density from resistance in nV/√Hz
- k = Boltzmann’s constant 1.38 x 10-23 J/K
- Tk = Temperature in Kelvin
- R = The input resistance referred to the non-inverting terminal of the amplifier
 Figure 1-1 Voltage Noise Density Curve
Figure 1-1 Voltage Noise Density CurveFigure 1-1 displays the relationship between noise spectral density (in nV/√Hz) and resistance (in ohms) plotted for T = 25°C (298K) with varying source resistance values. At just 1k ohms of source resistance the voltage noise is already at 4nV/√Hz.
It’s important to note that an ideal resistor will exhibit predictable noise density that is flat across the frequency spectrum. Multiplying Equation 1 by the noise bandwidth yields RMS. The noise bandwidth is the bandwidth of your circuit. This bandwidth can either be set by the operational amplifier internal circuitry or by using a filter. This RMS noise calculation is shown in Equation 2.

2 Operational Amplifier Voltage Noise
An operational amplifier has a voltage noise and current noise source. The magnitude of the noise sources inside the amplifier is given in the amplifier’s data sheet. When considering the voltage noise of an amplifier, it is important to realize the architecture of the amplifier. Typically, a bipolar input amplifier will have much lower voltage noise than a CMOS input amplifier for the same amount of quiescent current. For more information on the difference between amplifier architectures see this technical article: Trade-offs Between CMOS, JFET, and Bipolar Input Stage Technology. Before discussing the different types of voltage noise, it’s important to understand what this noise looks like in an amplifier circuit. Amplifier noise can be simplified by modeling it as an external voltage noise en(v) on the positive terminal of the amplifier as shown in Figure 2-1.
 Figure 2-1 Voltage Noise
Figure 2-1 Voltage NoiseAmplifier voltage noise can be broken down into two main components: flicker noise and broadband noise. Figure 1-1 displays these noise regions.
 Figure 2-2 Voltage Noise Density
Curve
Figure 2-2 Voltage Noise Density
Curve2.1 Flicker Noise
Flicker noise, or 1/f noise, is considered to be in the low frequency range; that is, frequencies less than 1kHz. 1/f noise has a slope of one divided by the square root of frequency. For low-frequency focused circuits, such as a woofer or bass control stage, 1/f noise can be critical. However for a circuit that covers the full audio bandwidth, the 1/f noise will not be a dominant noise contribution.